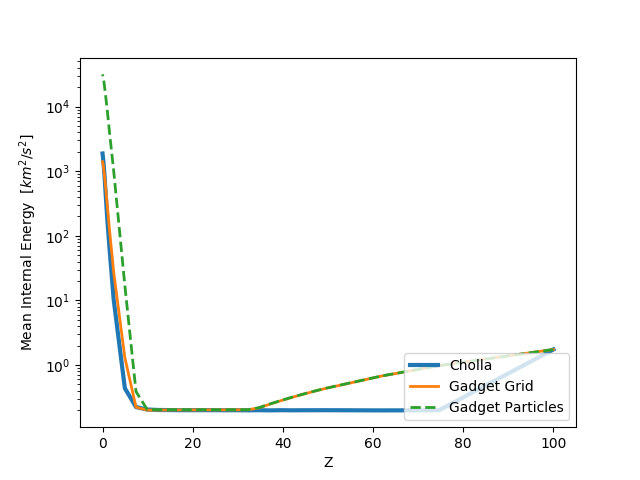
CHOLLA_PM Test: Gas Internal Energy and Power Spectrum (UPDATE)
Physical mean Internal Energy for early redshift
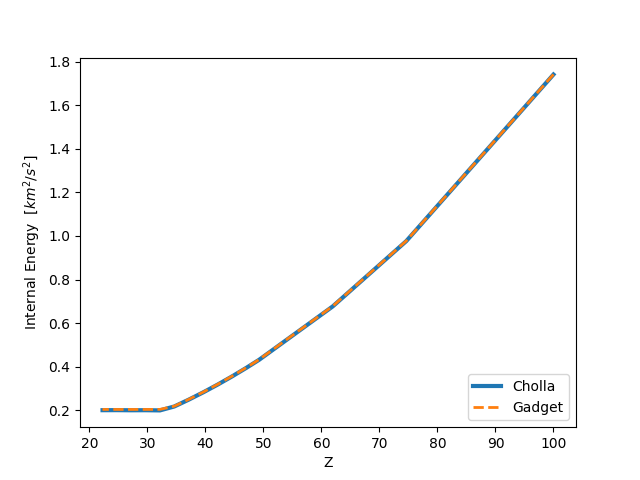
CASE 1: Zero initial velocities, no gravity acting on the gas
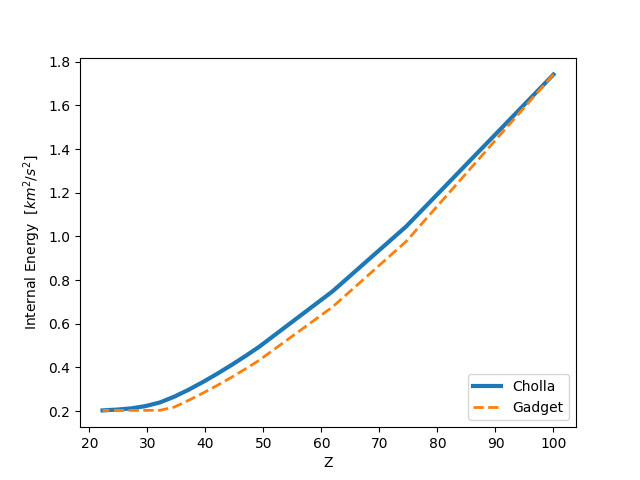
CASE 2: Initial velocities, NO gravity acting on the gas
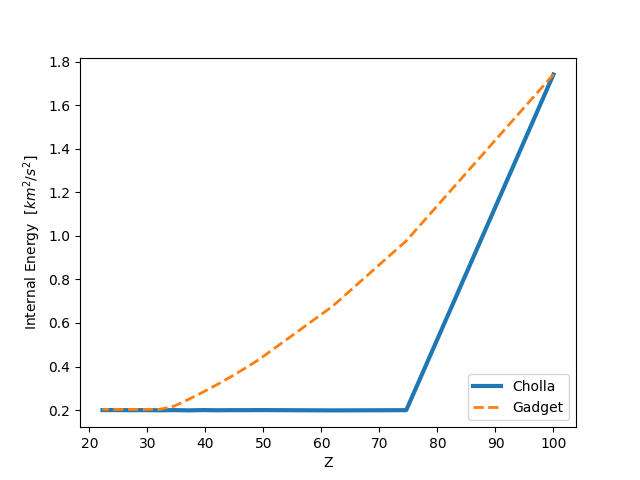
CASE 3: Initial velocities, Now gravity is acting on the gas, and the gas cools very fast.
From Initial Conditions
Dens mean: 13.4874
U mean: 0.00017072 \(km^2/s^2\)
Ek mean: 0.15950561 \(km^2/s^2\)
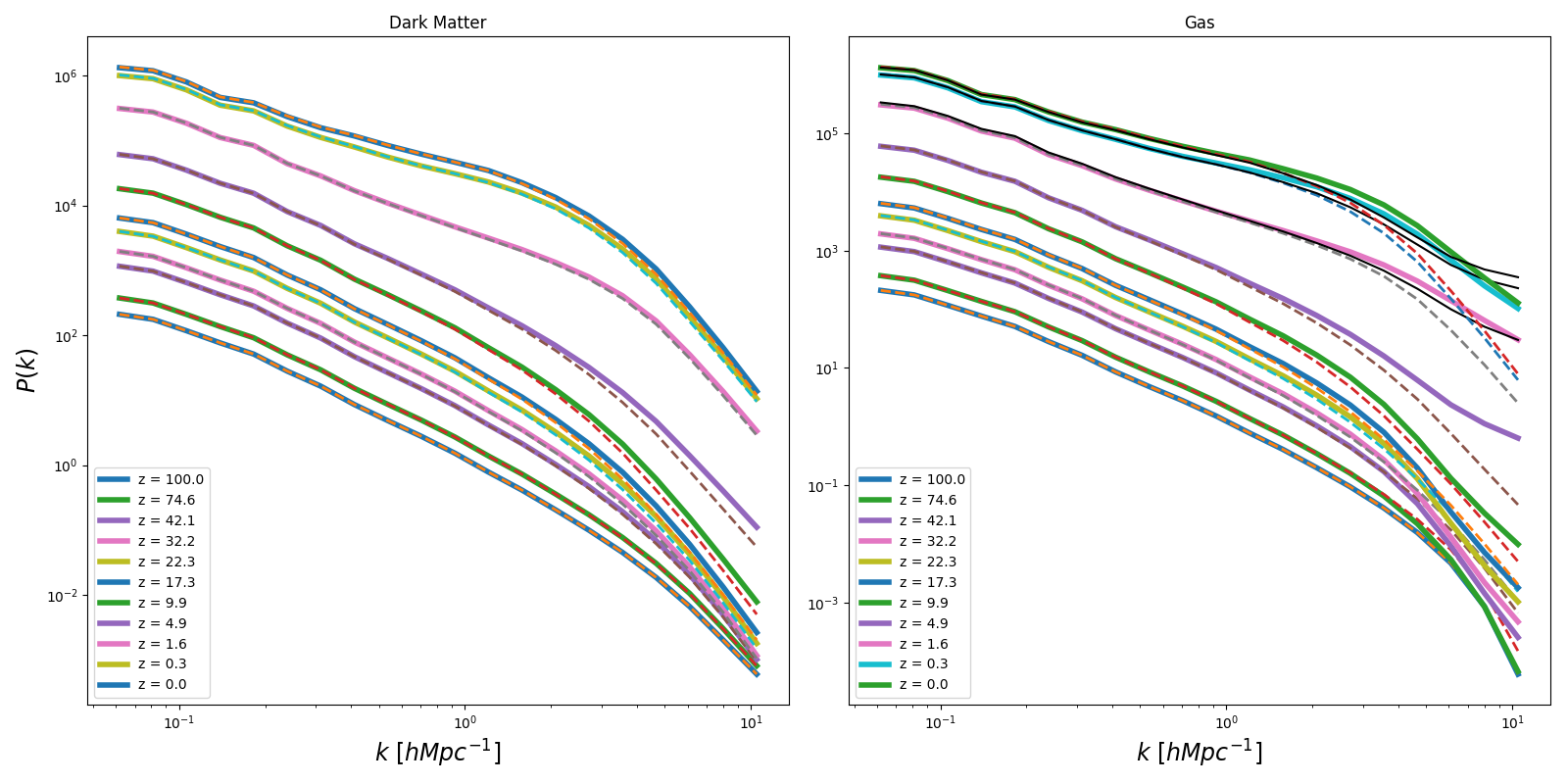
Physical mean Internal Energy for the entire simulation
Density Power Spectrum (update)
PREVIOUS: For this case the gravitational force acting on the Kinetic Energy of the gas was computed from the Virtual Work done by the gravitational force \(\Delta E = dt (-\rho \nabla \phi \cdot \overrightarrow{v})\)
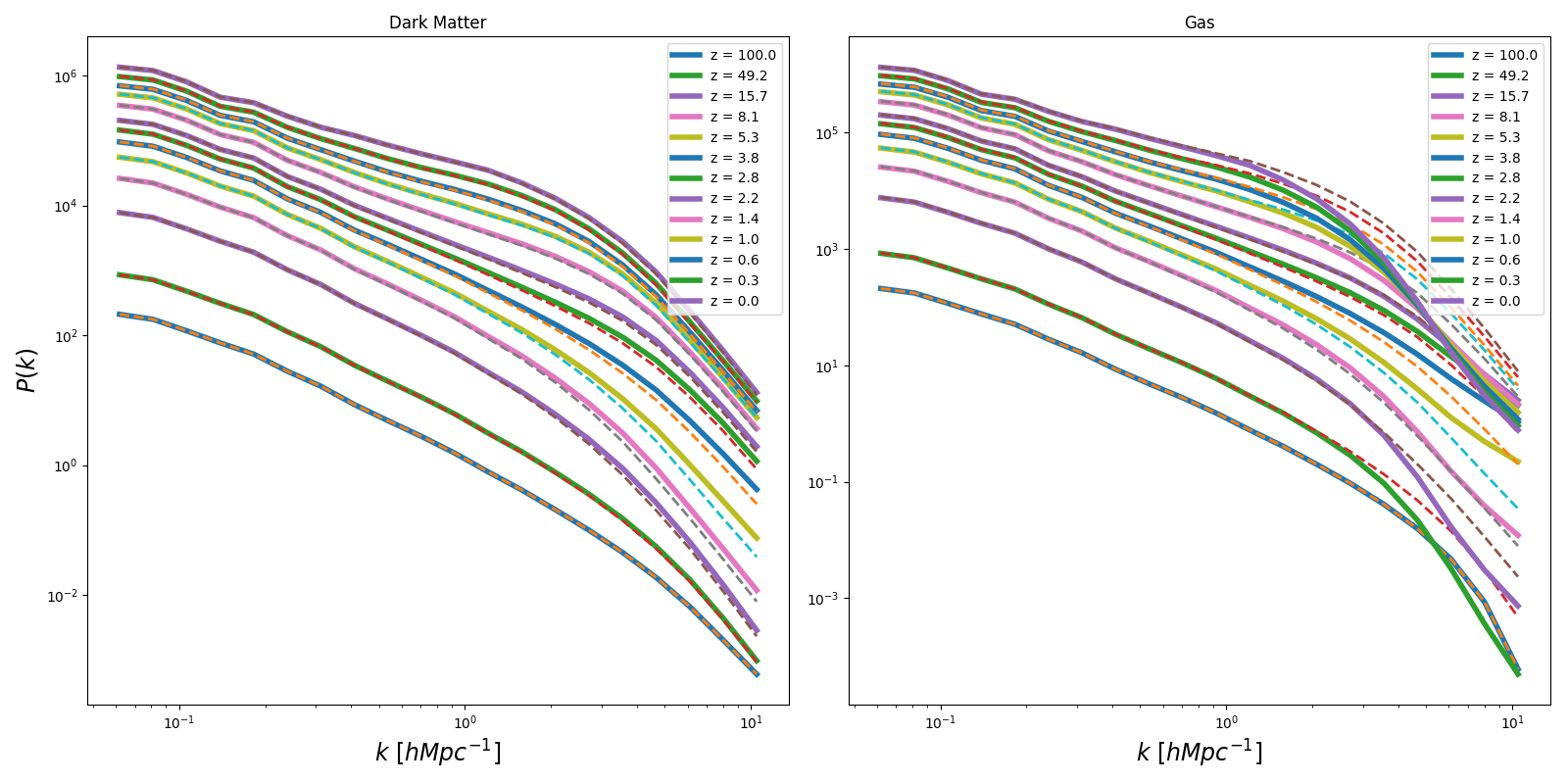
NOW: Instead of using the virtual work, I compute the change in momentum and use it to compute the change in Kinetic Energy \(\Delta E= dt( \Delta (P^2) / ( 2\rho ) )\)